Geology: What is Ore? Crustal Abundancies, sampling theory, and project complexity

Hello and welcome to episode 34 of The Con. My name is Merlin Marr-Johnson and in the previous episode I introduced a number of general geological concepts that are useful for understanding economic geology. The plan for today is to address the question of “What is Ore?”

Once this philosophical concept has been addressed I will have a look at the background crustal abundances of a variety of elements (and minerals) and then I’ll go on to remind you how rare and special it is for economic orebodies to form. Economic orebodies are rare and special because concentration factors are required to lift a mass of rock from levels of background crustal abundancies to anomalously high concentrations that can be defined and extracted at a profit. It takes a kind of geological magic to create “ore”, and I’ll illustrate a range of different ore styles and how they are developed.

Most deposits are hard to find, and when you come to think of it, measuring anything is actually hard to do as well. I’ll jump down the rabbit hole of sampling protocols and sample representativity, and with a bit of luck I’ll manage to navigate back out again into daylight.

The development of every ore deposit offers differing types of practical challenge. Some are more complex than others, requiring more advanced skillsets and, crucially, stronger balance sheets for success to be achieved. We know from experience, often bitter, that different types of orebodies have different Capital Expenditure requirements, and different barriers to entry. The last part of today’s episode will be a review of which kinds of companies are suited to which kinds of deposits, complete with gross generalisations and sweeping statements.

That is what I hope to cover today. End of the preamble. Let’s get stuck into the main topic.

Ore

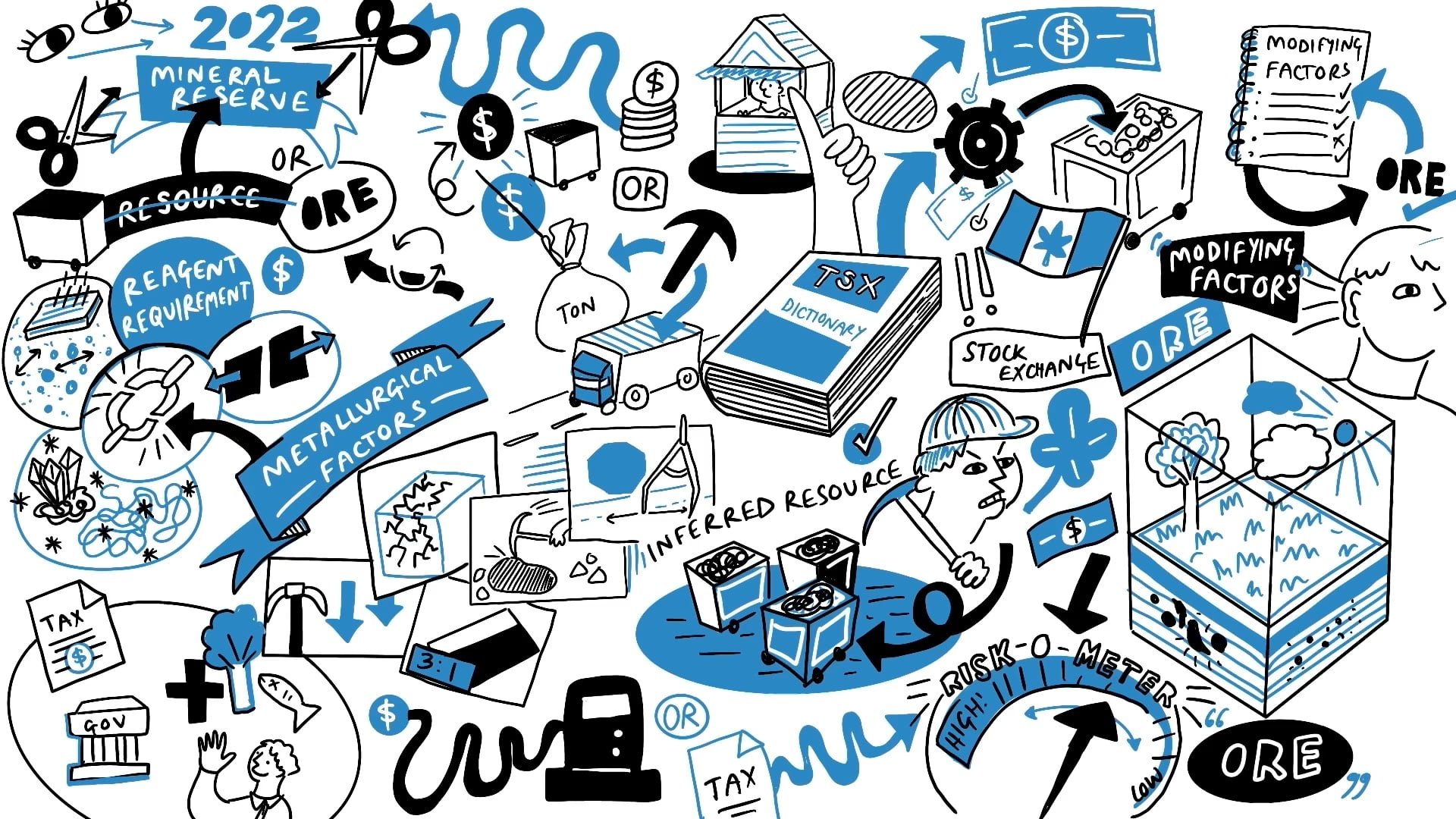
Ore, O,  R,   E,  is what the resources sector is all about. Define ore then dig it up at a profit, that’s the basic plan in a market economy. Ore is the stuff that gets mined, right? Perhaps; but getting there is not always simple, and there are hidden depths to that phrase ‘define ore’.

Starting from the principle that we know why we want use a certain commodity, exploration geologists often start with moose pasture and concepts; greenfields exploration and plenty of blue sky. With sufficient hard work, funding, and luck the team will find enough rock containing enough of the value material in question for it to qualify as an inferred resource. Eventually that resource might be derisked enough for it to be defined as ore. Regular listeners to The Con will have heard me use the term Modifying Factors that are applied to resources, to de-risk them further.  The Canadian stock exchange is explicit about the word “ore”. Under the TSX definition, “ore” implies technical feasibility and economic viability that should only be attributed to mineral reserves.

In other words, ore is only ore when a whole host of Modifying Factors have been satisfied so that the extraction of a valuable product from within that bit of rock can be done at a profit in a market economy. These Modifying Factors cover, at their most basic, geological factors such as grade, tonnage, and distribution; mining factors such as geometry, rock hardness, fracture patterns and or stability, and strip ratios or mining depths; metallurgical factors such as liberation and separation characteristics, reagent requirements, grind size, and mineralogical complexity; plus economic, political, environmental and social factors just for starters.

Fluctuating fuel prices, or tax rates, or commodity prices or reagent prices can make previously uneconomic resources economic. A resource can be redefined as a mineral reserve, or ore, under favourable conditions. But it cuts both ways. A change in the modifying factors can render ore uneconomic. And we are seeing this happen in 2022. In some cases material that last year was defined as Ore has now been downgraded to resource status as projects have been made uneconomic by rising capital and operating costs. Back to the drawing board for recently-but-no-longer bankable projects such as the Hemerden tungsten mine in Devon.

Hmmm. As you can see, defining Ore as ore is complicated. And yet, although the strict definition of Ore is wrapped up in the project specific detail, over time empirical observations can be made about different kinds of economic resources. And it is this aspect of ore bodies that I want to talk about next.

Different types of Ore

By and large, we have an idea of what tons and grades work for different kinds of deposits. Exploration companies set themselves loose discovery targets to guide fieldwork. Successful operations tend to meet certain threshold criteria and we can build up a picture of what works in real life situations. These criteria of course vary from company to company, from country to country, and from commodity to commodity, but they are used as guidelines. For example, Randgold Resources (before its merger with Barrick) used to target at least 2 million ounces at a grade of 2 grams per tonne. Rio Tinto now has its own framework of what kind of deposits meets Minimum Economic Value thresholds. When I spoke to a senior BHP geologist recently he said their target copper deposit is one that can produce 600,000 tonnes of copper per annum and at a minimum grade of 0.5% Cu. Not so easy to find! And so on. I spoke with a fund manager recently who had the view that copper porphyry resource grades needed to increase by a percentage point in grade from 0.4% in Arizona to 0.5% in Chile and Peru, and 0.6% in Argentina and Ecuador. Clearly metallurgy, strip ratio, tax rates and many other factors will affect project-specific economics, but empirical ideas of what constitutes an orebody exist.

Antony Evans wrote an excellent textbook called “Ore Geology and Industrial Minerals: An Introduction”. I’ve got a copy of the 3rd edition dating from the early 1990s, and it is a good book to own as a reference for anyone interested in the topic, even if it is a bit dated in places. In the introductory chapter, figure 1.17, there is a representation of the continuity of different orebody types and approximate grades.

see diagram / pdf / photo

Have a look at this diagram. The vertical axis shows concentrations ranging from 1 part per million (1ppm), 1g/t up to 100% pure mineral. The horizontal axis shows geological continuity ranging from high to low. Just as a reminder, 10,000 ppm is the same as 1%. 1,000 ppm is 0.1%. 100 ppm is 0.01%, etc. Remember, also, that there are a million grams in a metric tonne, so 1 ppm is also the same as 1g/t.

It is a diagrammatic representation of the continuity of different orebody types and approximate grades, dating from a paper written in 1982.  At the top of the diagram, the lowest grade deposits are diamond pipes, and then alluvial Gold, then it is a short hop to 30 ppm, or 30 g/t. In that very small range now sit most gold and platinum group metal deposits, with different levels of geological continuity from stratiform to vein-type. Any time there is a significant structural overlay on your basic orebody type, ore discontinuity will rise. The less geological continuity you’ve got, the harder it is to define your ore pods, the more the grade has to play in your favour.

Once the concentration, or grade, reaches 100 parts per million some deposits start to be measured in percentage terms. Lithium and uranium deposits are sometimes reported in ppm, so they don’t look so “low grade”. Molybdenum and cobalt, since they typically occur associated with copper or nickel, are usually reported in percentage terms.

The range for this low grade suite of minerals as shown here on the Y axis is 0.1% to 1%, or 1000 to 10,000 ppm. It’s a schematic diagram and we’re entering into the range of large porphyry deposits. Depending on a host of modifying factors, economic grades and commodity types, the deposits are going to be anything between 0.1% and 1%. The global grade of Copper being mined at the minute is around 0.45% Cu, which is just above the ‘average cut-off’ grade of copper deposits when the book was published about 30 years ago.

Rising in grade, with above 1% up to 10% of the rock mass comprised of value minerals, and you’re looking at nickel and tin sulphides.  Mississippi Valley-type VMS style deposits, stratiform hosted and volcanic-hosted Copper, Zinc, Lead, +/- Gold Silver deposits. These deposit types are usually smaller and more discontinuous than the big porphyry deposits and because they are more erratically in place, you’ve got to have higher grades to make them work. There are a host of metallurgical reasons and other modify factors as well but essentially, you’re talking about a grade that is roughly 10 times higher than the porphyry grades.

Taking another step higher in grade, one comes to the sedimentary Manganese and the Phosphorites at around 30%. At 40% - 50%, there are Bauxites and the majority of the Iron ore formations. Up at 60% content, there is some Podiform Chromite. Finally, with the value mineral comprising the vast majority of the rock itself there are Gypsum, Stratiform Chromite, Coal, and Salt. These are commodities that are potentially in situ materials that if you’ve got the right purity, you can sell them as you mine them - the fabled Direct Shipping ore.

The reason for showing this diagram is to remind you that ore comes in very very many different grades and types. The value mineral in a diamond deposit is almost unmeasurably scarce, and conversely in a salt deposit almost the entire rock is the value mineral. The challenges associated with defining and extracting these different deposit types vary enormously. For example, one of the challenges of low grade deposits is that a vast percentage by mass or volume of the ore is non-value mineral which needs to be mined, processed, and stored safely and the tiny percentage by mass or volume of the value-mineral needs to pay for the entire process. At the other extreme, huge amounts of rock need to be transported so logistics, handling, and distance to market become a key factor, and very small percentages of non-value minerals, deleterious elements or other impurities can affect the economics of the project.

Concentration Factors

Right. Having established that there is huge variability in actual ore types, now let’s look at concentration factors that are needed to be achieved to reach these broad grade thresholds.  How many times does the background level of mineralisation that’s the average endowment of the earth’s crust need to be concentrated to make an economically viable ore deposit? As a starting point, here are the crustal abundances of some key metals.

Look at Copper, a key mineral, for example. To reiterate, it has an average crustal abundance of 0.0068% or 68 parts per million. This means that to reach the average porphyry grades of around 0.3% to 0.5% Copper, nature has to cause an enrichment of 44 to 74 times the average crustal abundance. And the higher grade the deposit, the greater the enrichment or concentration factor required. 5% copper ore? You’re looking at concentraction factors of 740 times. And the same goes for all of those metals. Gold needs to be concentrated 250 x above crustal background to reach a grade of just 1 gram per tonne, 500 x to reach a grade of 2 g/t , 2500 x for 10 gram rock, and so on.

How does this happen? What causes ore grade material to occur? Well, I will cover the main processes of enrichment and concentration that can happen in geology in a separate talk about mineral systems. The key point I wanted to highlight here is that exploration and development companies are searching for the rare occurrences where natural processes have elevated, enriched, and concentrated the background mineralisation many many times over, and created unique locations where rock can be extracted as ore, for economic profit. Next time you berate exploration geologists for taking too long to find a deposit, or you rail at a deposit being small or low grade, it may be worth remembering the enormity of what nature has to do to make that deposit large and / or high grade.

Pierre Gy’s Theory of Sampling

At this juncture I would like to take a little time out to consider the concepts behind ascertaining grade. I mean, when we take a sample of something, how do we know what grade it is?  What if the point that is sampled does not have an ‘average’ amount of ore in it? I mean, we don’t sample 1 tonne of rock to establish how many grams of gold are contained within that tonne. No, we sample a couple of kilograms of rock and then scale up the proportion of gold found. But how does that work, huh? Well, the theoretical and practical science behind sampling owes a huge amount to a remarkable French metallurgist called Pierre Gy.

It all began in 1947 when Gy was working at a small lead (Pb) mine in the Republic of Congo and he was asked to estimate the grade of a 200,000 t, apparently low-grade, stockpile.  He soon recognised that fragments on the stockpile varied in size and weight, ranging from boulders of several tonnes to fine dust weighing almost nothing, and that he did not know what to do. Two key questions arose, which were: “How should one select a representative sample? and How much material should be selected for this purpose?” It was the starting point of the journey to an awesome set of Theory of Sampling monographs and papers that were published regularly from 1960 to 1998. Gy’s work incorporated statistical and stochastical theory, Bernoulli Trials, Poisson Sampling (which are all worth looking up if you have the inclination) and applied these ideas to problems such as sample heterogeneity, and variability, sampling errors and analytical errors. Snubbed by academia, misunderstood by his employers he carried out research by himself, earned not one but two PhDs in statistics along the way, and he ultimately established a formula to define a quantitative measure of heterogeneity, which “lies at the root of all sampling errors”. His work encompasses how to sample dynamic data sets (process plant flow, conveyor belts etc) as well as static data sets (laboratory analysis and ore deposits). Total genius!

To illustrate some of the challenges, imagine, if you will, ore with the value mineral disseminated finely and homogenously through a rock mass of a defined size, let’s call it the Lot. Let’s pretend the Lot contains gold. At pretty much every scale of sampling an even population within the Lot you would find the same proportion of gold to host rock. Even with a grade as low as, say, 1 g/t which is 1 part per million, in a perfectly homogenous sample the gold would be evenly distributed, so that if you sample it with a teaspoon, a ladle or a bucket, you would still find the same proportion of gold to host rock. But what happens if the distribution of the gold within your Lot is not even?

To make this point, let’s head into the kitchen and the glorious British tradition of Christmas pudding.

Mrs. Cratchit entered: flushed, but smiling proudly: with the pudding, like a speckled cannon-ball, so hard and firm, blazing in half of half-a-quartern of ignited brandy, and bedight with Christmas holly stuck into the top.

A Dickens scene. Well, the tradition also involves hiding a silver coin in the pudding. The aspiring exploration geologist can serve out 11 platefuls of pudding and analyse them each separately to come to the conclusion that this particular deposit had zero silver grade. And then a final serving, portion #12, which contains a coin….would show a different result. A naive geologist who was sitting by himself on Christmas Day, watching James Bond re-runs with this particular plate might think that the grade of this deposit is represented by the grade of this portion, so a grade of 1 silver coin per plate. Hurrah, we’ve made a high grade discovery! Let’s put out a sensational news release and claim success!

Not so fast. A wiser geologist would realise that she needs to take more samples to be sure, and would eventually reach the conclusion that the actual grade of this deposit is 1 silver coin per pudding. Hmm, maybe these puddings might not be worth mining after all.

Or imagine a huge muffin baked with only one blueberry for every million parts of cake. If you stuck a straw through that muffin to extract a core of tasty stuff, and then assessed the contents of the straw, you’d stand a good chance of thinking that you had intersected plain muffin rather than blueberry muffin, no? But if you ate the whole muffin you would realise that it is, in fact, a blueberry muffin.

The size of the sample required to ascertain the grade of any particular commodity will always be case specific. Care needs to be taken to ensure that individually, and collectively, samples adequately reflect the distribution of the value mineral under investigation. Bog this up, and you create a huge mess.

Stepping out of the larder and back into the field, gold might occur in nuggets, or preferentially in narrow quartz-veins that make up a tiny proportion of the overall rock mass. If you sample with a teaspoon, you may well miss the gold nugget or gold-bearing vein. Indeed, you might miss certain features even if you sample at the bucket-scale. There are a couple of ways round this conundrum, one is to take more samples in the hope that statistically speaking enough of the samples will contain the key features. The other method is to increase the volume of each sample taken. At the top end of this process of increasing sample size, “Bulk samples” are large samples of several tonnes or possibly hundreds or thousands of tonnes specifically planned and taken to tackle issues of heterogeneity.

Note that so far all we have been talking about is in-situ heterogeneity. Like a first-time mother fixated on the challenge of giving birth, once you’ve cracked that first problem there is no time for complacency. The next set of challenges come thick and fast. Once the rock has been broken or milled there is consitution heterogeneity caused by varying physical properties of the constituent parts, often density. For example, Gold (density 19.6 g/cm3) is 6-7x more dense than ‘average rock’ (density about 2.8 g/cm3) and gold will work its way to the lowest crevice very quickly.

Another food analogy. Have you ever opened a box of breakfast cereal and thought, yippee, this cereal has got loads delicious dried strawberries in it? And then realised that it was just the first bowlful and that every bowl after that was just the same old flakes as before? Did you infer the grade of the cereal from bowl one, or the larger sample size of all of the bowls around the breakfast table for as long as the cereal lasted? There you go. All this time you didn’t realise as you chomped your crispy flakes that you were dealing with a classic constitution heterogeneity problem! Incidentally, if there are family arguments over parity of dried strawberry distribution in bowls Pierre Gy can offer some practical steps in how to reduce the sample size without creating a systematic bias… Simply pour the entire packet of cereal onto the kitchen table, mix and then form it into a conical shape prior to flattening it out into a cake. Then divide the cake into quarters; remove the two quarters which sit opposite one another, combine the other two quarters which now constitute the reduced sample. And repeat the same process until you arrive at a breakfast portion sized sample. The dried strawberries will now be fairly distributed in every bowl. But you might have created a mess and solved one family argument only to create the grounds for another…

In short, there are in-situ problems and subsequent handling problems.

Taking a peek into the dark trunk that is the Theory of Sampling can be a bit scary. Very quickly the language becomes so bewilderingly complex, that it starts to sound comical to the layman. Sampling Errors can be Correct, Incorrect, Fundamental, or Total for starters, and there’s a veritable alphabet-soup of acronyms, even before the maths begins. But it is important, my goodness it’s important. Gy’s Theory of Sampling (TOS) is there, underpinning the entire industry.  It’s there in the way we take samples, in the number and the size of individual samples. If you look up the Australian JORC Code 2012, there is a huge section on ‘Sampling Techniques and Data’. In fact, Appendix 1 to the Code is all about sampling size, sampling techniques, and sampling protocols. The Theory of Sampling is there in everything that relies on using sample data. The JORC Code goes on to add that Sampling Techniques, I quote, “apply and should be considered when estimating and reporting Exploration Results, Mineral Resources and Ore Reserves”. In otherwords, the Theory of Sampling applies to pretty much everything we do as resource geologists.

Infill drilling and metallurgical sampling are effectively exercises in increase the volume and number of samples taken to reduce errors of estimation of average grade and to better understand any internal heterogeneity of the overall sample Lot. And remember that every meter of drilling, whether it is Reverse Circulation or Diamond Drillcore generates several kilos of sample. In the case of RC drilling, the sample comes up as dust and chips, and Diamond core often comes up in lumps or rock that may split preferentially along veins or in fault zones that are often fluid conduits and enriched in the value mineral. The samples all need to be reduced in size - in a representative manner - until they are small enough to be sent off to the assay lab, often a pulp as low as 85 grams in weight. Fire assay charges are typically 30 g or 50 g in weight, so an 85 g pulp can be reassayed and have some spare pulp left over. And each of those sub-samples need to be an accurate reflection of the original sample’s make-up.  Gy’s TOS is there in every step of the analytical process and the interaction of exploration companies with assay laboratories.

Yes, I know, on the scale of my usual disgressions this has been an elephant, but I feel it is important for you to know that science to calculate the grade of a sample is both well-established and sophisticated. This stuff matters, even if it rarely receives proper airtime. The grades of individual samples contribute to our understanding of the average grade for a mineral deposit, and it feeds into the process of Resource Estimation and the determination of Mineable Tonnes, and we are back full circle at the definition of Ore. Cool huh!?

At least now we can pause for breath. Hmmmmmm - and breathe.

Right. So far today I have talked about the Concept of Ore, differing grades of orebodies, the concentration factors required to acheive those ore grades, and the theoretical and practical challenges of sampling.

I did want to carry on to do two more topics but I realise now that my time is up, so I’m going to create a new Con which is going to come shortly after this one. And it will contain Basic Ore Types and Emprical Observations on project complexity. For now, however, goodbye.

CON 34 b

Hello and welcome to Episode 34b of The Con. This episode will cover Basic Ore Types and Empirical Observations on Project Complexity.

Ore Types

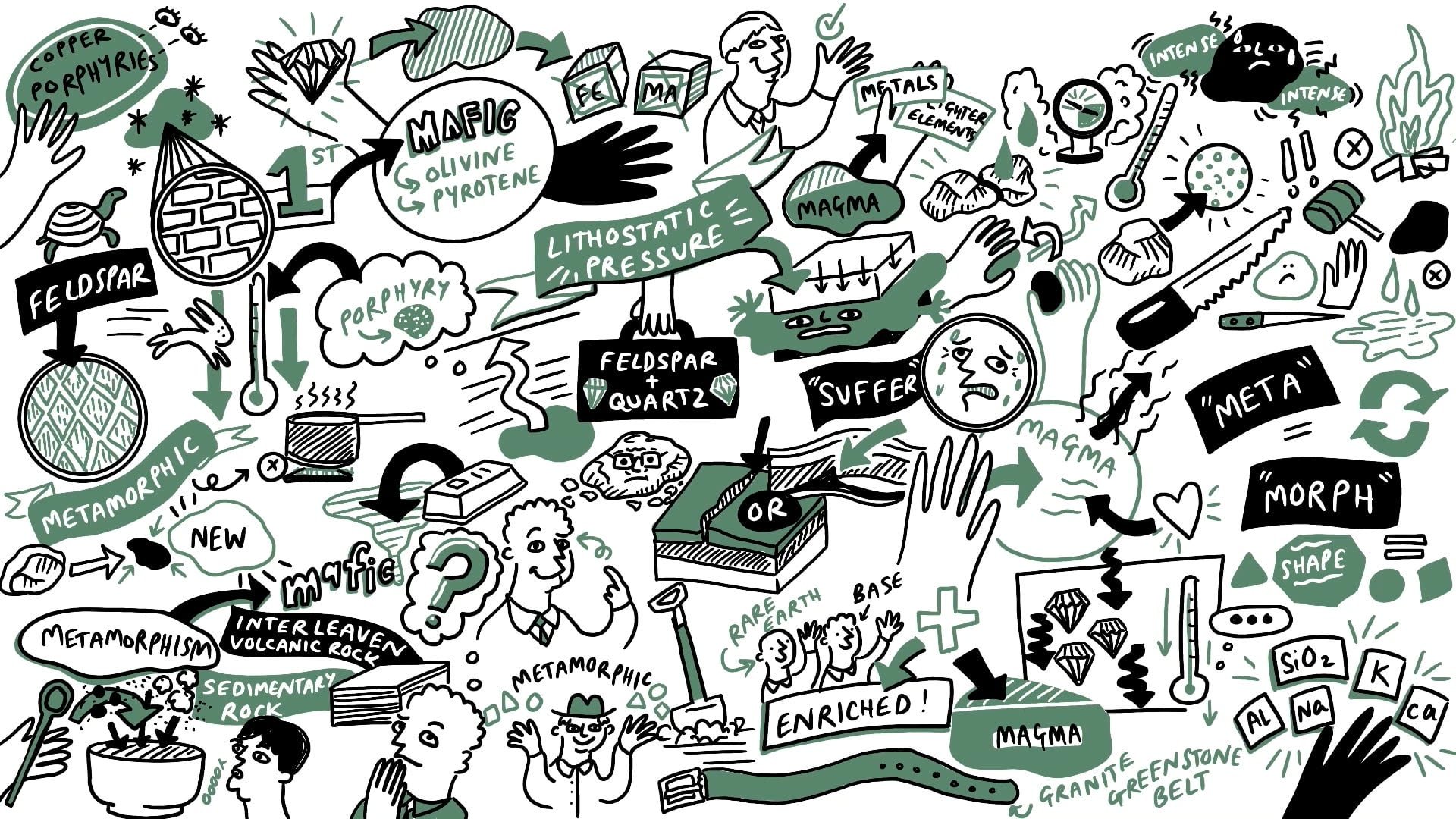
Mineral deposits can be categorised into three basic groups depending on how they are formed.

The first major group of ore-deposits are those created by the concentration, or enrichment of the value mineral through surficial processes such as weathering or sediment transport. Rock containing low concentrations of say, gold or diamonds, can be weathered (frost, rain, wind action), eroded, transported, and concentrated by river currents or wave action in the sea to form placer deposits. The less resistant rock is broken down into clay minerals over time, and washed or blown away at various stages during the processes. Ultimately the dense, resistant mineral (gold or diamonds) can either accumulate in-situ, or be transported by a high energy flow like a river, only to be preferentially trapped and concentrated in pockets (after waterfalls) or when the water loses energy such as when it flows out of the mountains onto the plains, or when it hits the sea.

Beach sands can form when resistant titanium minerals such as zircon, rutile and ilmenite are concentrated by the winnowing action of waves over thousands and thousands and thousands of years.

Bauxite, aluminum ore, is formed when surface waters preferentially remove other elements during weathering, leaving a rock that is, eventually, rich enough in aluminium to be mined economically.

The second major group of ore types are Magmatic Sulphides, that purely involve molten rock, or magma.  Magmatic Sulphides form through a process called sulphide unmixing. Normal magma is made of silicate minerals (rich in silica, aluminium, and a host of other rock-forming elements), but what can happen is that a separate melt of sulphide can form. Under certain conditions the sulphide separates out, so you end up with two melts, the silcate melt and a dense sulphide melt. And certain elements that really like to bond with sulphur move in the sulphide melt preferentially and get concentrated in that process. Cast your mind back to the different groupings on the Periodic Table - even if you can’t remember which elements go where - you can probably still picture in your minds’ eye that there were different groups. Well, each of those groupings is a set of elements with similar reactivities and chemical characteristics…. such as, yep, you guessed it, which elements like to bond with sulphur during geochemical processes. And if you have spent some time in the resources sector you will know that the big magmatic sulphide complexes such as Norilsk, Voisey’s Bay, Duluth, Stillwater, or the Bushveld Igneous Complex are famous for producing nickel, copper, cobalt, platinum, palladium, rhodium and other platinum group metals. [Note the use of the word ‘group’ there - PGMs or Platinum Group Metals - a nod back to Mendeleev and the Periodic Table]. This link between the geological setting and the production suite is because nickel, copper, cobalt and the PGMs preferentially partition into sulphide melts out of silicate melts in magmatic processes. When the sulphide binds with the metals and crystallizes it can form an ore that will have a metallic lustre, a steely or golden coppery colour.

Finally, the biggest and most diverse type of ore deposits are those formed by hydrothermal processes. These deposits are formed by the movement of water in the earth’s crust, through permeability pathways such as cracks, faults, or preferential fluid flow through certain strata. The water is usually hot - hydro, water…thermal, heat… - sometimes up to magmatic temperatures of around 600oC although ore-forming processes can occur at temperatures all the way down to relatively low temperatures. It goes like this - in certain conditions metals can dissolve in the water, move through the fluid conduits, and be deposited when the water can no longer hold the metal in solution. As I tell my children, if you’re not part of the solution, you’re the precipitate.  Anyway, a whole host of factors determine how the metal is dissolved; and precipitation occurs when there is a triggering change in one or more of Pressure, Temperature, or Chemistry of the fluid. If the trigger is very efficient or repeated multiple times in the same spot, then these hydrothermal processes can form ores.  More on this in the next episode, The Con #35.

The final section on Ore in this episode of The Con is based on Empirical rather than Scientific evidence. I will divide up deposit types into their suitability for development by different groups or business entities. What follows are gross generalisations and sweeping statements that may cause loathing and opprobrium to be heaped upon me, but I stand by the observations. I think the classification is rooted in common sense.

Complexity in mineral deposits and their economic development can come in many guises, such as low grade, difficult geometry, depth of burial, grade heterogeneity, mineralogical complexity, challenging process requirements, refractory minerals, high altitude, political instability, security concerns, transport and logistics bottlenecks and challenges, unstable ground, water management issues and flooding risk, temperature extremes, handling issues, ore variability, waste management, deleterious elements… the list goes on. Ultimately complexity is reflected in the management skills and the capital required to develop the asset. More complex, and generally speaking Bigger projects, demand higher capital expenditure requirements and higher management demands. Less complex, and generally speaking smaller projects demand less capital and managment skill. But remember that all projects are difficult and that it is easy to stuff up even theoretically simple projects. The resources sector has an unenviable track record of failure - most projects are late and over-budget (especially when compared to the initial capital estimates from the first ‘Feasibility Study’).

The easiest, lowest capital projects are suitable for consortiums, family businesses, start-ups and juniors. These are typically small or medium sized, metallurgically simple operations where nature has winnowed, oxidised, sorted, and weathered the rock to leave a minimal processing requirement. Included in this category are sand, gravel or dimension stone operations; alluvial projects - gold, PGMs, diamonds; tailings-retreatment projects, and oxide gold operations. Low capex, with simple metallurgy these operations can be strong cash flow generators. Crucially, when mistakes are made - as they inevitably will be - they are usually not too expensive to fix. Downsides to these kinds of operations are scale (they’re often small), growth challenges (tailings dumps are finite, oxide gold only persists to limited depth, etc), and resource definition challenges (placer deposits are notoriously fickle beasts to drill out and quantify).

Mid-capex projects are suitable for more experienced or ambitious groups. These deposits are moderately complex, with challenges that can be related to geology, metallurgy, mining technique, scale or logistics or a mix of all the above. Included in the category are sulphide gold deposits, silver-lead-zinc deposits, high grade copper, heap leach copper oxide, high grade nickel, small Direct Shipping Iron ore projects, or mineral sands projects. Teams need prior experience and balance sheet strength to tackle any of these projects successfully.

High capex projects are only suitable for major groups. These deposits are highly complex, usually very large scale, and can often involve commodities that are not traded on terminal markets. The risks associated with a junior company tackling these kinds of projects are unattractively high. Deposits in this category include coal, large DSO iron ore projects, low-grade copper-gold deposits such as porphyries or IOCGs, low grade nickel especially nickel laterite, hard rock diamond mines, beneficiated iron ore such as magnetite, platinum group metals, the rare earths suite, phosphates and fertilizers, and large-scale uranium.

A rule of thumb is that engineers should not run projects with capital estimates 1 & 1/2 times larger than the previous project they ran. The same could be said for Companies. Complexity and Cost are real barriers to entry.

Goodness, it has been a long episode and we’ve covered a lot of ground. In the next session I will talk about the six key components that need to be in place in a Mineral System for deposits to be formed. Until then, thank you for listening, and goodbye.

‍